
HEAT
We spend considerable sums of money every year to buy heat. We buy it in the form of coal, gas and electricity for the house, and as petrol for the car. How much heat can be obtained from 1 ton of coal or from 1 unit of electricity? The scientist must know these figures if he is designing heating equipment. But no figures can be given until a unit of heat has been chosen.
SIMPLE EXPERIMENT ON HEATING
Take a beaker with 400 с. с. (or 400 gm.) of water in it and heat it with a bunsen flame. Place a thermometer in the water and record the temperature every minute. Stir the water with the thermometer continuously. Stop when a temperature of 60° С is reached. Repeat the experiment in a similar beaker, use the same bunsen flame, first with 200 gm. of water and then with 200 gm. of paraffin oil. Record the results of each experiment in a table.
Since the same flame has been used throughout the experiment, we can assume that the rate at which heat is received in each case is constant. After examining your table you will find:
a) The rise in temperature is twice as fast with the 200 gm. of water as it is with the 400 gm. of water.
b) In each case the rate of rise of temperature is constant: the temperature of the 400 gm. of water rises at a constant rate and the increase per minute is the same; the rise in temperature per minute of the 200 gm. of water is again constant but it is twice that of the 400 gm. of water.
We may now conclude that the quantity of heat necessary to raise the temperature of a substance:
1. is proportional to the mass of the body;
2. is proportional to the rise in temperature. Finally if we examine the results for paraffin oil, we see that the weight of the oil is 200 gm. but the temperatures do not correspond to the temperatures for 200 gm. of water. The paraffin oil has a rate of rise of temperature almost twice that for the same weight of water. We can add a third conclusion:
3. The quantity of heat necessary to raise the temperature of a substance depends upon the nature of the substance. It means that it varies for different substances.
Now we may measure the quantity of heat produced in a given time by a bunsen burner. But first we must decide what will be our unit of heat.
UNITS
As we have seen that the rise in temperature depends on the nature of- the substance and on its mass, we must specify these two quantities and the actual rise in temperature if we wish to define our exact quantity of heat. We choose the substance which . is cheap and easily obtainable in a very pure form. So we choose water. And for the mass we select unit mass. And for the rise in temperature 1 degree.
Metric System.
One calorie is that quantity of heat which will raise the temperature of 1 gm. of pure water through 1 deg. C. It is written as 1 cat.
The calorie is a small quantity of heat and it is convenient to have a larger unit. Such a unit is the kilo-calorie or just Calorie written with a capital C.
A kilo-calorie is the quantity of heat which will raise the temperature of one kilogram of pure water through 1 deg. C. 1 kilo-calorie or 1 Calorie = 1000 cat.
beaker мензурка
is twice as fast with в два раза быстрее при
is twice that of в два раза больше
1 cal. = 1 calorie 1 калория
table таблица
HEAT GAINED AND HEAT LOST
From the definition of the calorie we see that:
The temperature of 1 gm. of water will be raised 1 deg. C. by 1 cal.
Thus the temperature of 5 gm. of water will be raised 1 deg. C. by 5 cal. and the temperature of 5 gm. of water will be raised 6 deg. C. by 5 × 6 cal. =30 cal.
Thus the heat necessary to raise the temperature of some water is given by the product of the mass of the water and its rise in temperature.
Heat gained by water = Mass × Rise in temperature.
Similarly we can say that the heat lost when water cools is the product of the mass of the water and its •fall in temperature.
Heat lost by water = Mass × Fall in temperature.
We shall now consider an experiment to find the effect of mixing different masses of water at different temperatures.
Experiment.
Heat 40 gm. of water in a beaker to about 50° С. In a second beaker place 60 gm. of water which will be at room temperature. Note each temperature carefully. Quickly pour the hot water into the cold water. Stir with the thermometer and quickly note the maximum temperature.
SPECIMEN RESULTS
Weight of hot water - 40 gm.
Weight of cold water - 60 gm.
Temperature of hot water - 50° C. (fifty Centigrades)
Temperature of cold water - 15° C.
Temperature of mixture - 28° C.
Fall in temperature of hot water - (50 - 28) deg. C. = 22 deg. C. and Rise in temperature of cold water - (28 - 15) deg. C. =, 13 deg. C.
Heat lost by hot water in cooling = Mass × Fall in temperature = (40 × 22) cal. = 880 cal. and Heat gained by cold water = Mass × Rige in temperature = (60 × 13) cal. = 780 cal.
The hot water has therefore lost more heat on cooling than the cold water has gained. In our experiment exactly 100 cal. have been lost. Where has the heat gone? Some of it has been lost to the air as the hot water was transferred to the cold water. If we examine our experiment carefullv, we see that the beaker containing the cold water will also have its temperature raised from 15° C. to 28° С. and the missing heat will be used in raising the temperature of the beaker through this range.
SPECIFIC HEAT
Our experiment showed us that the rate of rise of temperature of the paraffin oil was about twice that of water. Then if one calorie of heat raises the temperature of 1 gm. of water 1 deg. C., it will raise the temperature of 1 gm. of paraffin oil about 2 deg. C.
For our work in heal we must know the quantity of heat which will raise the temperature of 1 gm. of any given substance through 1 deg. C. This quantity is called the specific heat of the substance.
The specific heat of a substance is the number of calories of heat necessary to raise the temperature of 1 gm. of it through 1 deg. C.
We will prove later that the units of specific heat are cat. per gm. per deg. C.
Suppose we denoted the specific heat (S. H.) of a substance as s cal. per gm. per deg. C. Then we can say: the quantity of heat to raise 1 gm. of the substance through 1 deg. C. = 1 s cal;
the quantity of heat to raise 4 gm. of the substance through 1 deg. C. = 4 s cal.;
the quantity of heat to raise 4 gm. of the substance through 10 deg. C. - 40 s cal.
Finally we can say that the heat required to raise any mass of a substance through any temperature range is given by the product of three things:
Quantity of heat, required = Mass × S. H. × Rise in temperature.
If Q is the quantity of heat in calories to raise m gm. of a substance of specific heat s through t deg. C., then Q = M ×S × t cal.
If the substance is cooling, the heat lost is given by the same equation, though t is now the fall in temperature.
We can now find the units in which specific heat is measured.
Q is measured in calories;
m is measured in grams;
t is measured in degrees Celsius.
Therefore if we put these units in the equation Q =m × s × t, we get cal. = gm. × 5 × deg. C.
To Find the Specific Heat of a Substance
Some methods of finding the specific heat of a substance are too difficult but can be performed.
Experiment.
The specific heat of glass. We know that 100 cal. of heat are needed to raise the temperature of the beaker from 15° С. to 28° С. Weigh the beaker dry. Let it weigh 48 gm. Then the specific heat of glass may be calculated as follows:
By definition, the S. H. of glass is the quantity of heat necessary to raise the temperature of 1 gm. of glass through 1 deg. C.
From our experiment we have:
The temperature of 48 gm. of glass is raised through 13 deg. C. by 100 cal;
the temperature of 1 gm. of glass is raised through 13 deg. C. by
| 100 | cal; |
| 48 |
the temperature of 1 gm. of glass is raised through 1 deg. C. by
| 100 | cal; |
| 48 × 13 |
∴ the S. H. of glass is 0.16 cal. per gm. per deg. C. (50 - 28) deg. C. is read fifty minus twenty-eight degrees of Celsius.
per gm. - per gram на грамм
per deg. C. = per degree of Celsius на градус Цельсия
through 1 deg. С. = Is cal. is read through one degree of Celsius equal s calories.
the heat required to тепло, требуемое для того, чтобы
Q = m × s × t cal. is read Q equals m by s by t calories let it weigh пусть он весит
the S.H. = the specific heat удельная теплоёмкость
| 100 | cal; |
| 48 |
is readone hundred forty-eights (в простых дробях числитель читается, как количественное числительное, а знаменатель - как порядковое числительное, т. е. как в русском языке: и т. д.).
The Effect of the High Specific Heat of Water on Climate
It is well known that islands such as the British Isles have a more equable climate than places at equal latitude situated in large continental land masses. Let us consider an example. The British Isles will have an average annual range of temperature of about 15 deg. F. and places on the same latitude in Siberia may experience an annual range of as much as 95 deg. F.
One important reason for this difference is the high-specific heat of water which is about five times as great as that of earth, rock or sand. Owing to its high specific heat the water heats more slowly than the land and the temperature of the sea in early summer will be less than the temperature of the coast. This will cause cool breezes to blow from the sea over the land reducing its temperature. In winter the oceans are a vast reservoir of heat so that winds blowing over the sea are warmed and moderate the climate of any coast line which they meet.
The influence, of the oceans is particularly noticeable when we come to the two poles of the Earth. The North Pole is in the middle of a sea, but the South Pole is in the middle of a continent with an area larger than the area of Europe. The South Pole is far colder than the North. At the South Pole the average yearly temperature over the vast continent is from 10 to 20 degrees F. below zero.
annual годовой
F. - Fahrenheit Фаренгейт
far colder намного холоднее
is about five times as great as that of earth почти в пять раз больше, чем удельная теплоемкость земли
pour лить, вливать
reservoir резервуар
HOW HEAT TRAVELS
If a pot of fresh tea stands for a long time, it loses heat. We say heat is lost, or is transmitted to the surroundings. If we stand the pot on a cork mat and cover it with a tea-cosy, it still loses heat, but more slowly. The rate at which heat is transmitted to the surroundings has been reduced. Heat is transmitted from a hot body, the tea-pot, to the surroundings which have a lower temperature. The rate at which heat is transmitted from a hot body to a cold one depends upon what lies between the two bodies.
There are different ways by which heat may be transmitted from one point to another. One of them is known as conduction.
To Compare Different Metals as Conductors of Heat
Some metals are much better conductors of heat than others, a fact which may be shown by the following experiment using heat-sensitive paper. This is prepared by soaking a sheet of white cartridge paper in a solution of cobalt chloride. When the paper is slowly but thoroughly dried, it retains its white colour. If, however, any portion of the paper is heated, it turns a light blue colour. On cooling in a moist atmosphere the original white colour is restored.
The marks on the paper show that heat has been conducted much farther along the copper than along either of the other two. We conclude that copper is the best conductor of the three and that brass is better than iron.
Good and Bad Conductors of Heat
Solids are the best conductors of heat, liquids are much poorer and gases do not conduct heat at all. Even solids differ greatly in their ability to conduct heat. Silver is the best conductor, copper is almost as good. Such metals as iron and lead are far behind, but all metals are considered good conductors when they are compared with such solids as wood or glass.
Many solids are used for their power of conducting heat well or because they are bad conductors.
Good Conductors - the Davy Lamp
In 1812 92 men and boys were killed in an explosion in the Felling Mine in England. This was caused by the explosive nature of a mixture of air and firedamp (methane), a gas often present in mines. So long as miners used naked candle flames such explosions often occurred. After the disaster Humphry Davy was asked to construct a lamp which would give adequate illumination for the miner and prevent explosions. He carried out a series of experiments which convinced him that the answer lay in the conducting power of a copper gauze. Let us repeat such an experiment.
Experiment.
Place a copper gauze about 2 in. above the top of a bunsen burner. Turn on the gas and light it above the gauze. The gas burns freely above the gauze but shows no tendency to ignite beneath it (Fig. a). An inflammable gas or vapour will ignite only if it is raised to a certain temperature called its ignition point. The copper gauze is a good conductor, and conducts the heat away from the flame so rapidly that the gas beneath the gauze never reaches its ignition point. For the same reason if the gas is lit beneath the gauze, no flame will appear above it (Fig. b).
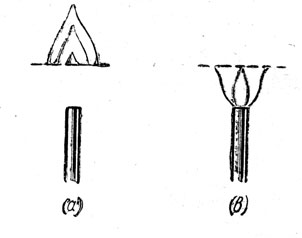
Fig. (a), (b)
Humphry Davy applied this principle in his safety lamp. The flame from the oil lamp is surrounded first by thick glass through which its light shines. Above the glass is a cylindrically-shaped copper gauze. The heat from the flame rises into the area surrounded by the gauze which conducts it away so efficiently that the explosive mixture of firedamp and air on the outside never reaches its ignition temperature. The air passes into the lamp and, if firedamp is present, a blue cap is seen on top of the flame. This acts as a warning to the miner that this dangerous gas is present. Although it is burning inside the lamp, sufficient heat can never get to the outside to cause a general explosion. Nowadays a miner carries an electric lamp, but, in order to give a warning of the presence of firedamp, a Davy lamp is usually carried in addition by the leader of each group of workers.
Bad Conductors and their Uses
There are many uses for bad conductors of heat. Often we wish to prevent heat passing from a warm body to its surroundings, or to stop heat from outside passing to a cooler body. Substances which will do this are often called heat insulators. In order that their varied uses may be studied, we shall place our bad conductors in three groups.
| Group I | Group II | Group III |
| Cork | Wool | Air |
| Wood | Flannel | Asbestos |
| Bone | Fur | Vacuum |
| Glass | Feathers | - |
| Rubber | Nylon | - |
| Foamed plastic | - | - |
bunsen burner бунзенская горелка
cartridge paper плотная бумага (для рисования и для патронных гильз)
cobalt chloride хлорид кобальта
cork mat подставка из пробки
cylindrically-shaped в форме цилиндра
either of the other two зд. по любому из двух других
firedamp рудничный газ, гремучий газ
heat-sensitive paper теплочувствительная бумага
tea-cosy чехол на чайник
|
ПОИСК:
|
При использовании материалов сайта активная ссылка обязательна:
http://genling.ru/ 'Общее языкознание'